| |
|
Abstract: |
If
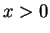 ,
is a Hardy-type operator defined on the cone of monotone functions, then weight
characterizations for which the modular inequality ,
is a Hardy-type operator defined on the cone of monotone functions, then weight
characterizations for which the modular inequality
holds, are given for a large class of modular functions  .
Specifically, these functions need not both be .
Specifically, these functions need not both be 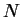 -functions,
and the class includes the case where -functions,
and the class includes the case where 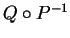 is concave. Our results generalize those in [7,24], where the case
is concave. Our results generalize those in [7,24], where the case 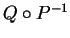 convex, with
convex, with  , , 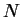 -function
was studied. Applications involving the Hardy averaging operator, its dual, the
Hardy-Littlewood maximal function, and the Hilbert transform are also given. -function
was studied. Applications involving the Hardy averaging operator, its dual, the
Hardy-Littlewood maximal function, and the Hilbert transform are also given.
[7] P. DRÁBEK, H.P. HEINIG AND A. KUFNER, Weighted modular inequalities for
monotone functions, J. of Inequal. and Appl., 1 (1997), 183–197.
[24] J.Q. SUN, The modular inequalities for a class of convolutions operators on
monotone functions, Proc. Amer. Math. Soc., 125 (1997), 2293–2305.
|



