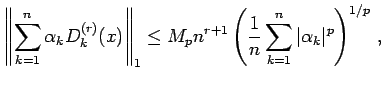JIPAM

On an Bojanic-Stanojevic type inequality  
 
  Authors: Zivorad Tomovski,  
  Keywords: Bojanic-Stanojevic inequality, Sidon-Fomin's inequality, Bernstein's inequality, $L^1$-convergence, cosine series.  
  Date Received: 22/09/99  
  Date Accepted: 07/03/00  
  Subject Codes:

26D15,42A20.

  
  Editors: Hari M. Srivastava,  
 
  Abstract:

An extension of the Bojanic-Stanojevic type inequality [1] is made by considering the $ r$-th derivate of the Dirichlet kernel $ D_{k}^{(r)}$ instead of $ D_{k}$. Namely, the following inequality is proved

$displaystyle leftVert sum_{k=1}^{n}alpha _{k}D_{k}^{(r)}(x)rightVert _{1... ...{r+1}left( frac{1}{n}sum_{k=1}^{n}vertalpha _{k}vert^{p}right) ^{1/p} ,$    

where $ Vert cdot Vert _{1}$ is the $ L^{1}$-norm,$ {alpha_{k}}$ is a sequence of real numbers, $ 1pleq 2$, $ r=0,1,2,ldots $ and $ % M_{p}$ is an absolute constant dependent only on $ p$. As an application of this inequality, it is shown that the class $ mathcal{F}_{pr}$ is a subclass of $ mathcal{B}mathcal{V}cap mathcal{C}_{r}$, where $ mathcal{F}_{pr}$ is the extension of the Fomin's class, $ mathcal{C}_{r}$ is the extension of the Garrett-Stanojevic class [8] and $ mathcal{B}mathcal{V}$ is the class of all null sequences of bounded variation.

[1] R. BOJANIC and C.V. STANOJEVIC, A class of L1-convergence, Trans. Amer. Math. Soc., 269 (1982), 677-683.
[8] Z. TOMOVSKI, An extension of the Garrett- Stanojevic class, Approx. Theory Appl., 16(1) (2000) 46–51. [ONLINE] A corrected version is available in the RGMIA Research Report Collection, 3(4), Article 3, 2000. URL: http://rgmia.vu.edu.au/v3n4.html ;


This article was printed from JIPAM
http://jipam.vu.edu.au

The URL for this article is:
http://jipam.vu.edu.au/article.php?sid=107