| |
|
|
| |
Volume 10, Issue 1, Article 19 |
|
|
|
|
|
| |
Generalized $\lambda$-Newton Inequalities Revisited
|
| |
|
Authors: |
Jianhong Xu, |
|
| |
|
Keywords:
|
Elementary symmetric functions, $lambda$-Newton inequalities, generalized $lambda$-Newton inequalities, arithmetic mean-geometric mean inequality, positive stable matrices, determinant-trace inequality. |
|
| |
|
Date Received:
|
23/10/08 |
|
| |
|
Date Accepted:
|
10/02/09 |
|
| |
|
Subject Codes: |
05A20, 05E05, 15A15, 15A42, 15A45, 26D05
|
|
| |
|
Editors: |
Jerry J. Koliha, |
|
| |
|
|
|
|
|
| |
|
Abstract: |
We present in this work a new and shorter proof of the generalized 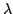 -Newton inequalities for elementary symmetric functions defined on a self-conjugate set which lies essentially in the open right half-plane. We also point out some interesting consequences of the generalized -Newton inequalities for elementary symmetric functions defined on a self-conjugate set which lies essentially in the open right half-plane. We also point out some interesting consequences of the generalized 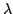 -Newton inequalities. In particular, we establish an improved complex version of the arithmetic mean-geometric mean inequality along with the corresponding determinant-trace inequality for positive stable matrices. -Newton inequalities. In particular, we establish an improved complex version of the arithmetic mean-geometric mean inequality along with the corresponding determinant-trace inequality for positive stable matrices.
|
|
|
|
|
|
| |
|
|
|
|
| |
|
|
|
|
|



