| |
|
|
| |
Volume 10, Issue 2, Article 53 |
|
|
|
|
|
| |
On Application of Differential Subordination for Certain Subclass of Meromorphically $p$-Valent Functions with Positive Coefficients Defined by Linear Operator
|
| |
|
Authors: |
Waggas Galib Atshan, S.R. Kulkarni, |
|
| |
|
Keywords:
|
Meromorphic functions, Differential subordination, convolution (or Hadamard product), $p$-valent functions, Linear operator, $delta$-Neighborhood, Integral representation, Linear combination, Weighted mean and Arithmetic mean. |
|
| |
|
Date Received:
|
06/01/08 |
|
| |
|
Date Accepted:
|
02/05/09 |
|
| |
|
Subject Codes: |
30C45.
|
|
| |
|
Editors: |
Sever S. Dragomir, |
|
| |
|
|
|
|
|
| |
|
Abstract: |
This paper is mainly concerned with the application of differential subordinations for the class of meromorphic multivalent functions with positive coefficients defined by a linear operator satisfying the following: In the present paper, we study the coefficient bounds, 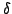 -neighborhoods and integral representations. We also obtain linear combinations, weighted and arithmetic means and convolution properties.
|
|
|
|
|
|
| |
|
|
|
|
| |
|
|
|
|
|
Home
