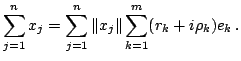JIPAM

Reverse Triangle Inequality in Hilbert $C*$-Modules  
 
  Authors: Maryam Khosravi, Hakimeh Mahyar, Mohammad Sal Moslehian,  
  Keywords: Triangle inequality, Reverse inequality, Hilbert $C*$-module, $C*$-algebra.  
  Date Received: 16/10/2009  
  Date Accepted: 13/11/2009  
  Subject Codes:

Pri: 46L08; Sec: 15A39, 26D15, 46L05, 51

  
  Editors: Sever S. Dragomir,  
 
  Abstract:

We prove several versions of the reverse triangle inequality in Hilbert $ C^*$-modules. We show that if $ e_1, dots, e_m$ are vectors in a Hilbert module $ {mathfrak{X}}$ over a $ C^*$-algebra $ { mathfrak{A}}$ with unit 1 such that $ langle e_i,e_jangle=0 (1leq i<br />eq j leq m)$ and $ Vert e_iVert=1 (1leq ileq m)$, and $ r_k,ho_kin mathbb{R} (1leq kleq m)$ and $ x_1, dots, x_nin {mathfrak{X}}$ satisfy

$ 0leq r_k^2 Vert x_jVertleq mathop{m Re}<br />olimits langle r... ...^2 Vert x_jVert leq mathop{m Im}<br />olimits langle ho_ke_k,x_j rangle ,$    

then
$left[ sum_{k=1}^mleft(r_k^2+ho_k^2ight)ight]^{frac{1}{2}}sum_{j=1}^n Vert x_j VertleqleftVertsum_{j=1}^nx_jightVert ,$    

and the equality holds if and only if
$ sum_{j=1}^n x_j=sum_{j=1}^nVert x_j Vertsum_{k=1}^m(r_k+iho_k)e_k .$    
;


This article was printed from JIPAM
http://jipam.vu.edu.au

The URL for this article is:
http://jipam.vu.edu.au/article.php?sid=1166