| |
|
|
| |
Volume 3, Issue 4, Article 53 |
|
|
|
|
|
| |
On the Sequence $(p_n^2-p_{n-1}p_{n+1})_{n\ge 2}$
|
| |
|
Authors: |
Laurentiu Panaitopol, |
|
| |
|
Keywords:
|
Prime Numbers, Sequences, Series, Asymptotic Behaviour. |
|
| |
|
Date Received:
|
17/12/01 |
|
| |
|
Date Accepted:
|
24/05/02 |
|
| |
|
Subject Codes: |
11A25,11N05,
|
|
| |
|
Editors: |
László Tóth, |
|
| |
|
|
|
|
|
| |
|
Abstract: |
Let 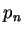 be the be the 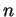 -th prime number and -th prime number and 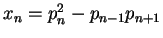 . In this paper, we study sequences containing the terms of the sequence . In this paper, we study sequences containing the terms of the sequence 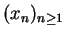 . The main result asserts that the series . The main result asserts that the series 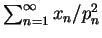 is convergent, without being absolutely convergent. is convergent, without being absolutely convergent.
|
|
|
|
|
|
| |
|
|
|
|
| |
|
|
|
|
|



