|
In this paper we denote by ![$ m_n^{[r]}$](images/227_04_JIPAM/img1.gif) the following expression, which is closely connected to the weighted power means of order the following expression, which is closely connected to the weighted power means of order ![$ r, M_n^{[r]}$](images/227_04_JIPAM/img2.gif) . . Let 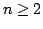 be a fixed integer and be a fixed integer and where 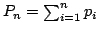 and and 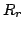 denotes the set of the vectors denotes the set of the vectors 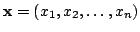 for which for which 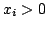 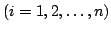 , , 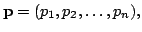 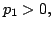 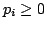 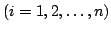 and and 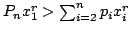 . . Three inequalities are presented for ![$ m_n^{[r]}$](images/227_04_JIPAM/img1.gif) . The first is a comparison theorem. The second and the third is Rado type inequalities. The proofs show that the above inequalities are consequences of some well-known inequalities for weighted power means. . The first is a comparison theorem. The second and the third is Rado type inequalities. The proofs show that the above inequalities are consequences of some well-known inequalities for weighted power means.
| 


