JIPAM logo: Home Link
 
Home Editors Submissions Reviews Volumes RGMIA About Us
 

   
  Volume 7, Issue 1, Article 17
 
On the Star Partial Ordering of Normal Matrices
    Authors: Jorma K. Merikoski, Xiaoji Liu,  
    Keywords: Star partial ordering, Normal matrices, Eigenvalues.  
    Date Received: 19/09/05  
    Date Accepted: 09/01/06  
    Subject Codes:

15A45, 15A18.

  
    Editors: Simo Puntanen,  
 
    Abstract:

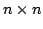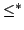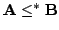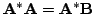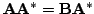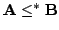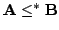We order the space of complex $ ntimes n$ matrices by the star partial ordering $ starleq$. So $ mathbf{A}starleqmathbf{B}$ means that $ mathbf{A}^*mathbf{A}=mathbf{A}^*mathbf{B}$ and $ mathbf{A}mathbf{A}^*=mathbf{B}mathbf{A}^*$. We find several characterizations for $ mathbf{A}starleqmathbf{B}$ in the case of normal matrices. 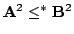As an application, we study how $ mathbf{A}starleqmathbf{B}$ relates to $ mathbf{A}^2starleqmathbf{B}^2$. 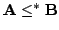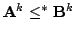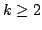The results can be extended to study how $ mathbf{A}starleqmathbf{B}$ relates to $ mathbf{A}^kstarleqmathbf{B}^k$, where $ kgeq 2$ is an integer.

         
       
  Download Screen PDF
  Download Print PDF
  Send this article to a friend
  Print this page
  

      search [advanced search] copyright 2003 terms and conditions login