| |
|
|
| |
Volume 7, Issue 5, Article 195 |
|
|
|
|
|
| |
On the Bounds for the Spectral and $\ell _p$ Norms of the Khatri-Rao Product of Cauchy-Hankel Matrices
|
| |
|
Authors: |
Hacı Civciv, Ramazan Türkmen, |
|
| |
|
Keywords:
|
Cauchy-Hankel matrices, Kronecker product, Khatri-Rao product, Tracy-Singh product, Norm. |
|
| |
|
Date Received:
|
21/07/05 |
|
| |
|
Date Accepted:
|
10/05/06 |
|
| |
|
Subject Codes: |
15A45, 15A60, 15A69.
|
|
| |
|
Editors: |
Fuzhen Zhang, |
|
| |
|
|
|
|
|
| |
|
Abstract: |
In this paper we first establish a lower bound and an upper bound for the  norms of the Khatri-Rao product of Cauchy-Hankel matrices of the form norms of the Khatri-Rao product of Cauchy-Hankel matrices of the form 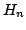 = = ![$ left[ 1/left( g+(i+j)hright) right] _{i,j=1}^{n}$](images/223_05_JIPAM/img3.gif) for for 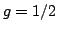 and and 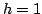 partitioned as partitioned as where 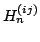 is the is the 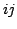 th submatrix of order th submatrix of order 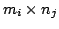 with with 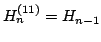 . We then present a lower bound and an upper bound for the spectral norm of Khatri-Rao product of these matrices. . We then present a lower bound and an upper bound for the spectral norm of Khatri-Rao product of these matrices.
|
|
|
|
|
|
| |
|
|
|
|
| |
|
|
|
|
|



