Review of the Book
Series Associated with the Zeta and Related Functions
By
H.M. Srivastava and Junesang Choi
Kluwer Academic Publishers, Dordrecht/Boston/London,
2001, ix,
pp. 388, ISBN 0-7923-7054-6
The Riemann Hypothesis (RH) concerning the zeros of the Riemann
Zeta function has had, and is continuing to have, a profound and
far-reaching effect on the activity of many mathematicians since
its enunciation by Riemann in 1859. At the 1900 address to the
Paris International Congress of Mathematicians, Hilbert listed the
RH as one of his famous list of 23 mathematical problems. It still
remains unsolved to this day despite the effort expounded to date.
In the quest for its solution it has developed many new areas of
mathematics because of its implication in the solution of many
problems (see for example, Conrey (2003) and Borwein et
al. (2000)).
The current book investigates an even more fundamental problem,
that relating to the summation of reciprocal powers of series
which are broadly termed as Zeta and related functions. The book
aims, with considerable success, in giving a unified and
systematic treatment of identities involving the Riemann zeta
function 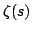 and related functions including; the Hurwitz
zeta
and related functions including; the Hurwitz
zeta
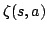 and Lerch's transcendent
and Lerch's transcendent
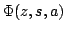 .
.
Some of the results stem from a 1729 theorem of Christian Goldbach
as reported in some correspondence with Daniel Bernoulli. The
theorem involves the closed form solution of
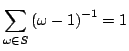 |
(1) |
where the sum is over omega belonging to the set 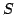 of all non-trivial integer kth powers. In terms of the Riemann
zeta function
of all non-trivial integer kth powers. In terms of the Riemann
zeta function 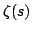 , Goldbach's theorem may be restated as
, Goldbach's theorem may be restated as
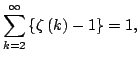 |
(2) |
where
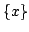 indicates the fractional part of
indicates the fractional part of
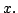
The book is aimed at providing a source of reference material for
researchers requiring results associated with the Zeta function
and related series. Detailed and methodical presentation of
techniques for handling zeta type series is given and placed in a
historical context giving the reader an appreciation of its
origins. An extensive list of references is provided together with
tables of identities demonstrating the wealth and depth of many
results of series and integrals satisfied by Zeta related
functions.
The book consists of six chapters each of which culminates with
generous sets of problems relating to the matter covered in the
chapters and reference is made to the original source of the
problems from which the interested reader may seek further
elaboration on the solution methodology.
The first chapter provides a very nice overview of some classical
functions and their properties which gives good background
material required for the subsequent chapters. It covers; Gamma,
Beta, Polygamma and Hypergeometric functions together with
Bernoulli, Euler numbers and polynomials and, Stirling numbers of
the first and second kind. Chapter 2 defines the zeta and related
functions and develops results related to those in Chapter 1 while
in Chapter 3 series involving the zeta function are evaluated
giving a very extensive list of result while in Chapter 4 the
emphasis is in providing representations in terms of fast
convergent series and investigating convergence properties of Zeta
functions in particular for the odd integers. In Chapter 5, the
computation of the determinants of the Laplacians on manifolds of
constant curvature is investigated which involves the closed form
evaluation of Zeta functions. The final Chapter 6 involves the
investigation of closed form evaluation of some of the special
functions defined in Chapter 1 in terms of Zeta related
functions.
I have a concern with the book and that is with the method of
referencing of equations in sections such as a.b(c) referring to
equation c in section a.b of chapter a. The equation numbering is
started afresh in each section although the top of the pages only
indicate the chapter so that one needs to find the start of the
section by going to the table of contents.
Overall, the book will serve as a very valuable reference
presenting and documenting an extensive array of both
methodologies and results involving the Zeta and related
functions. It is a worthwhile addition to the arsenal for
researchers interested in this area of analysis which has a very
long historical interest both in itself and in the extensive areas
of application.
P. Cerone
[1] J.M. BORWEIN, D.M. BRADLEY and R.E. CRANDALL, Computational strategies for
the Riemann zeta function,
Numerical analysis in the 20th Century, Vol. I, Approximation theory. J. Comput. Appl.
Math., 121(1-2) (2000), 247--296.
[2] J. BRIAN CONREY, The Riemann hypothesis, Notices Amer. Math. Soc., 50(3)
(2003), 341--353.




 Printable Version
Printable Version