Up: Table of Contents
Next: 1 Prelude: Gauss mappings of plane curves
Preface
In his Disquisitiones generales circa superficies curvas
(1827) [Gau], Gauss introduced the
spherical image of an oriented surface in space. Each point P
on the surface is mapped to that point Q on the unit sphere
whose radius vector is parallel to the outward normal vector to the
surface at P. The determinant of the derivative of the Gauss
mapping at P is the Gaussian curvature K of the
surface at P . If K(P) 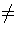 0, a small simple closed curve about P on the
surface is mapped to a simple closed curve about Q on the
sphere. These two curves will have the same orientation if
K(P) > 0 (P is elliptic), or
opposite orientations if K(P) < 0 (P is
hyperbolic). Gauss indicated that he intended to study the
geometry of the spherical image near a point P with
K(P) = 0 (a parabolic point), but he
never published anything about his investigations.
0, a small simple closed curve about P on the
surface is mapped to a simple closed curve about Q on the
sphere. These two curves will have the same orientation if
K(P) > 0 (P is elliptic), or
opposite orientations if K(P) < 0 (P is
hyperbolic). Gauss indicated that he intended to study the
geometry of the spherical image near a point P with
K(P) = 0 (a parabolic point), but he
never published anything about his investigations.
Hilbert and Cohn-Vossen discussed several examples of Gauss
mappings near parabolic points in their book Anschauliche
Geometrie (1932) [HC, 529]. The spherical image of the bell
surface has a "fold" along the image of the parabolic locus. The
parabolic locus of the torus of revolution is two circles, each
of which has a single point as spherical image. The monkey
saddle has an isolated parabolic point, which is a ramification
point of the Gauss map.
The Gauss map of the bell surface is stable: any
sufficiently small perturbation of the map has the same
topology. However, there is an arbitrarily small perturbation of the
Gauss map of the monkey saddle which has a curve as its parabolic
image, and which therefore has a different topological type. We shall
see that such a perturbation can be realized as the Gauss map of a
perturbed monkey saddle. This illustrates a theorem of Looijenga [Lo] and Bleeker and Wilson [BlW]: For an open dense set in the space
of immersions of a surface in 3-space, the Gauss map is stable.
Whitney [Wh] showed that the only local
singularities of a stable map of surfaces are folds and
cusps. (The map
(x, y) -> (x, y2)
has a fold singularity at
(x, y) = (0, 0), and
(x,y) -> (x, y3 - xy)
has a cusp singularity at
(x, y) = (0, 0).) The singularities
of the Gauss map of a surface are precisely the parabolic points. If
the Gauss map is stable, almost all of the parabolic points are fold
points of the Gauss map. In this paper we shall investigate the
extrinsic geometry of a surface at a point where the Gauss map has a
cusp. This not only puts classical geometric concepts in a new light,
but also vividly illustrates the powerful techniques being developed
in the theory of singularities of families of differentiable maps.
We obtain a list of ten geometric characterizations of cusps of Gauss
mappings, of which the following three are the most interesting.
Theorem. If the Gauss map N of the immersion X
: M2 -> R3 is stable,
then N has a cusp at P in M if and only if any
one of the following statements is true:
1) The restriction of X to arbitrarily small neighborhoods
of P has bitangent planes.
2) A ridge of X crosses the parabolic curve of X at
P, and the principal curvature associated to the ridge is zero
at P.
3) P is a parabolic point of X which is in the
closure of the set of inflection points of asymptotic curves of
X.
There are two types of Gaussian cusps: the elliptic cusp,
at which the projection of the surface to its normal line has an
extremum, and the hyperbolic cusp, at which this projection has
a topological saddle. Each of the above three characterizations of
cusps of the Gauss map reflects the difference between the two
types. For an elliptic [hyperbolic] cusp, the nearby bitangent planes
touch the surface at elliptic [hyperbolic] points. The line of
curvature associated to the zero curvature direction at an elliptic
[hyperbolic] cusp lies in the elliptic [hyperbolic] region. The
configurations of asymptotic lines which occur near an elliptic cusp
are topologically distinct from the configurations which occur near a
hyperbolic cusp.
Characterization (1) occurs in the work of Zakalyukin [Z], Romero Fuster [Rom1], and Bruce [Bru1]. Characterization (3) occurs in the
work of Arnold [A6], Kergosien and Thom [KeT] (see also [BaT]), Landis [Lan], and Platonova [Pl2]. Arnold has informed us that many of our
results are known to the Russian school of singularity theory
(cf. chapter 8 below). Much of their work is summarized in a
forthcoming book [AGV]. A detailed
investigation of cusps of Gauss mappings is also contained in the work
of Y. L. Kergosien [Ke1] [Ke2] [Ke3].
We would like to express our debt to the pioneering work of Rene
Thom on the geometry of singularities of differentiable maps [T1] [T2] [T4]. His description of focal surfaces using
catastrophe theory [T4, p. 96], as developed
by Porteous [Por1], was a starting point
for our research.
Several other applications of singularity theory to differential
geometry are discussed in Wall's survey "Geometric properties of
generic differentiable manifolds" [Wa2]
(cf. also [Bru2]). Many interesting ideas
and examples in this vein occur in an unpublished manuscript of
Michael Menn. An introduction to singularity theory is given by the
survey articles of Callahan [C1], Arnold [A1], Wall [Wa1],
and Levine [Le].
Our first chapter is about plane curves, where the interplay
between the singularities of the Gauss map and singularities of
families of functions takes on a very simple form. In the second
chapter we introduce five examples which we shall use to illustrate
our results. The singularities of the Gauss maps in these examples are
analyzed using techniques of Bleeker and Wilson [BlW]. A description of the remarkable
confluence of geometric properties which occurs at a Gaussian cusp
appears in the following chapter. The proofs that these properties are
equivalent for generic surfaces occur in chapters 4 through 7. We
conclude with a short chapter summarizing current work on singularity
theory and the extrinsic geometry of curves and surfaces.
The computer graphics illustrations in the original document [BaGM2] were created by Thomas Banchoff and
Charles Strauss. Their film, "The Gauss map, a dynamic
approach" includes our examples 2 and 3a (see [BaT]).
[Web graphics and mpeg movies by Dan Dreibelbis, using CenterStage and
StageManager,
external modules for Geomview. Images
of mathematical equations were created by latex2html.]
We thank Rick Porter for a stimulating lecture on envelopes of
plane curves and catastrophe theory which pointed out very fruitful
directions for this research project.
| Thomas Banchoff | Mathematics
Department
Brown University
Providence, Rhode Island
02912
U.S.A. |
| Terence Gaffney | Mathematics
Department
Northeastern University
Boston, Massachusetts
02115
U.S.A. |
| Clint McCrory | Mathematics
Department
University of Georgia
Athens, Georgia
30602
U.S.A. |
Supported in part by the National Science Foundation, Grants number
MC579-01310 and MC579-04905.
Up: Table of Contents
Next: 1 Prelude: Gauss mappings of plane curves
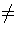 0, a small simple closed curve about P on the
surface is mapped to a simple closed curve about Q on the
sphere. These two curves will have the same orientation if
K(P) > 0 (P is elliptic), or
opposite orientations if K(P) < 0 (P is
hyperbolic). Gauss indicated that he intended to study the
geometry of the spherical image near a point P with
K(P) = 0 (a parabolic point), but he
never published anything about his investigations.
0, a small simple closed curve about P on the
surface is mapped to a simple closed curve about Q on the
sphere. These two curves will have the same orientation if
K(P) > 0 (P is elliptic), or
opposite orientations if K(P) < 0 (P is
hyperbolic). Gauss indicated that he intended to study the
geometry of the spherical image near a point P with
K(P) = 0 (a parabolic point), but he
never published anything about his investigations.