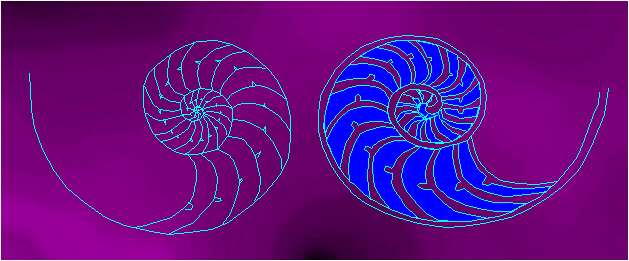

The idea of similarity symmetry and the possibility for its exact mathematical treatment was introduced in the monograph by H. Weyl (1952), who defines two similarity transformations of the plane E2: a central dilatation (or simply, dilatation) and dilative rotation, with the restriction for the dilatation coefficient k > 0, and he establishes the connection between the transformations mentioned and the corresponding space isometries - a translation and twist, respectively. His analysis is based on natural forms satisfying similarity symmetry (e.g., the Nautilus shell, Figure 3.1.; the sunflower Heliantus maximus, etc.). In considering a spiral tendency in nature Weyl quotes certain older authors (e.g., Leonardo and Goethe), who also studied these problems and also that of a phyllotaxis, the connection between the way of growth of certain plants and the Fibonnaci sequence, linked to a golden section (H.S.M. Coxeter, 1953, 1969). The sequence 1,1,2,3,5,8,13¼ defined by the recursion formula: f1 = 1, f2 = 1, fn+fn+1 = fn+2, n Î N, is called the Fibonnaci sequence. A golden section ("aurea sectio" or "de divina proportione", according to L. Paccioli) is the division of a line segment so that the ratio of the larger part to the smaller is equal to the ratio of the whole segment to the larger part, i.e. its division in the ratio t:1, where t is the positive root of the quadratic equation t2+t+1 = 0, t = (Ö5+1)/2 » 1,618033989...

|
Cross-section of a Nautilus shell. |
The next step in the development of the theory of
similarity symmetry in the plane E2 was a contribution by
A.V. Shubnikov (1960). He described all the similarity
transformations of the plane E2: central dilatation K,
dilative rotation L and dilative reflection M and the
symmetry groups derived by one of the transformations mentioned
and by isometries having the same invariant point - rotations
and reflections. Shubnikov derived six types of discrete
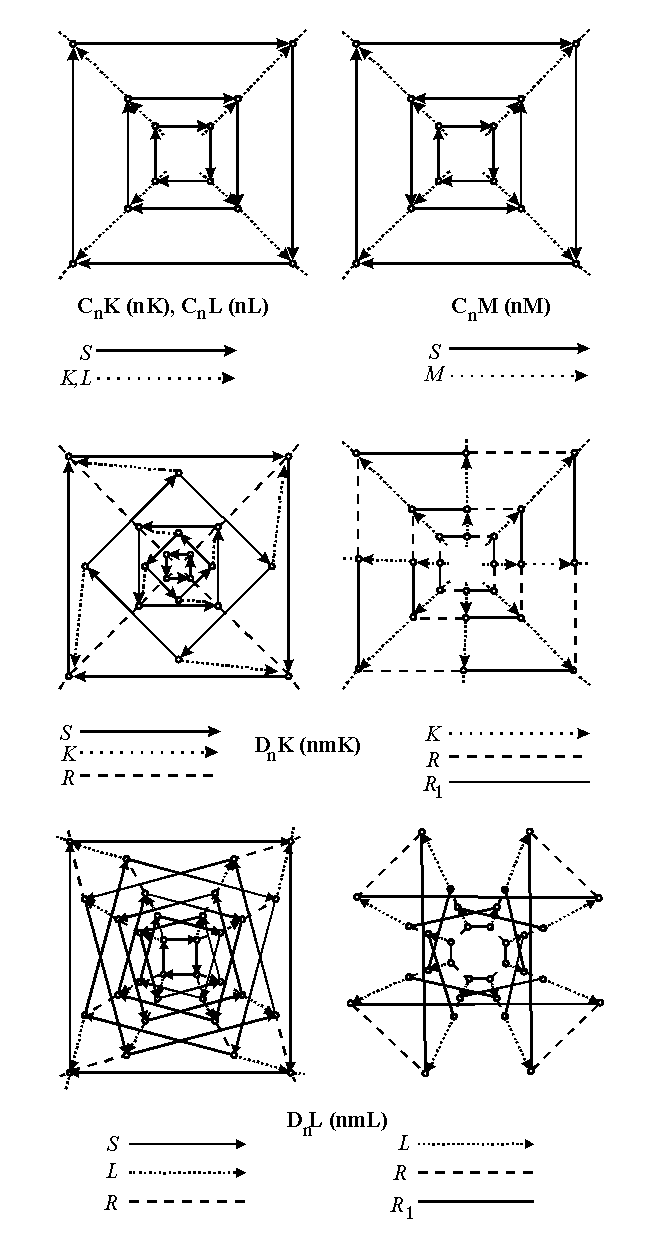
similarity symmetry groups of rosettes S20: CnK,
CnL, CnM, DnK, DnL,
DnM, denoted by Shubnikov nK, nL, nM, nmK,
nmL, nmM respectively. Since the types DnM
(nmM) and DnL (nmL) coincide, there
are, in fact, five types of the discrete similarity symmetry
groups of rosettes S20: CnK (nK),
CnM (nM), CnL (nL), DnK (
nmK), Dn L (nmL) and two types of the visually
presentable continuous similarity symmetry groups of rosettes
S20: D¥ K (¥K) and
CnL1 (nL1). The term "type of similarity symmetry groups of
rosettes" and the corresponding type symbol denote all the
similarity symmetry groups defined by this symbol, that can be
obtained by different combinations of parameters defining them.
For example, by the symbol CnK (nK) are denoted
all the corresponding similarity symmetry groups which can be
obtained for different values of n
(n Î N) and k
(where K = K(k)).
Presentations and structures:
CnK (nK) {S,K} Sn = E SK = KS Cn×C¥
CnL (nL) {S,L} Sn = E SL = LS Cn×C¥
CnM (nM) {S,M} Sn = E SMS = M
DnK (nmK) {S,R,K} Sn = R2 = (SR)2 = E
KR = RK KS = SK Dn×C¥
{R,R1,K} R2 = R12 = (RR1)n = E
KR = RK
KR1 = R1K
DnL (nmL) {S,R,L} Sn = R2 = (SR)2 = E
LS = SL
LRLR = RLRL RLR = LS
{R,R1,L}
R2 = R12 = (RR1)n = E
LRLR = RLRL
LR1LR1 = R1LR1L
R1L = LR (L = L2n = L(k,p/n))
Form of the fundamental region:
bounded, allows changes of the shape of
boundaries that
do not belong to reflection lines,
so symmetry groups of
the types CnK (nK),
CnL (nL),
CnM (nM)
allow changes
of the shape of all the boundaries, while symmetry groups
of the types DnK (nmK),
DnL (nmL) allow only
changes
of the shape of boundaries that do not belong to reflection
lines.
Number of edges of the fundamental region:
DnK (nmK) - 4;
CnK (nK), CnL (nL),
CnM (nM) - 4,6;
DnL (nmL) - 3,4,5,6.
Enantiomorphism: symmetry groups of the types CnK (nK),
CnL (nL),
CnL1
(nL1) give the possibility for the enantiomorphism.
In all other cases the enantiomorphism does not occur.
Polarity of rotations: coincides with the polarity of rotations
of the generating
symmetry groups of rosettes Cn (n), Dn (nm).
Polarity of radial rays: if they exist, radial rays are polar.
The table of group-subgroup relations between discrete similarity symmetry
groups of rosettes S20:
| CnK | CnM | DnK | DnL | |
| CnK | 2 | |||
| CnM | 2 | 2 | ||
| DnK | 2 | 2 | 2 | |
| DnL | 2 | 2 | 2 | 3 |
If q = pp/q, (p,q) = 1, then:
Further analysis on similarity symmetry groups was undertaken by E.I. Galyarski and A.M. Zamorzaev (1963). Besides giving the precise definitions of the similarity transformations K, L, M, they used the adequate names for these transformations, comparing them, respectively, with the corresponding isometries of the space E3 - translation, twist and glide reflection. They also successfully established the isomorphism between the similarity symmetry groups of rosettes S20 and the corresponding symmetry groups of oriented, polar rods G31. In this way, consideration of the similarity symmetry groups of rosettes S20 and their generalizations is reduced to the consideration of the corresponding, far better known symmetry, antisymmetry and color-symmetry groups of polar, oriented rods G31. The principle of crystallographic restriction (n = 1, 2, 3, 4, 6) is followed by E.I. Galyarski and A.M. Zamorzaev.
Isomorphism between similarity symmetry groups of rosettes S20 and symmetry groups of polar rods G31 is, according to A.V. Shubnikov and V.A. Koptsik (1974):
| CnK (nK) | (a)n |
| CnL (nL) | (at)n |
| CnM (nM) | (a)nã |
| DnK (nmK) | (a)nm |
| DnL (nmL) | (a)(2n)nm = (a)(2n)nã |
| D¥ K (¥mK) | (a)¥m |
| CnL1 (nL1) | (a)¥0n |
In the work by E.I. Galyarski and A.M. Zamorzaev (1963), there is no the restriction for the dilatation coefficient k > 0, used by H. Weyl (1952). This restriction does not result in any loss of generality, but only in the somewhat different classification of the similarity symmetry groups of rosettes S20.
There is also the problem that for every particular similarity symmetry group of rosettes S20, its corresponding type is not always uniquely defined. Namely, under certain conditions, the same symmetry group can be included in two different types. Such a case is, e.g., that symmetry groups of the type CnK (nK), because of the relationship K(k) = L(k,0), also belong to the type CnL (nL). If we accept the condition K = K(k) = L(k,0) = L0, then there also exists the subtype DnL0 (nmL0), but symmetry groups of the subtype mentioned are not included in the type DnL2n (nmL2n). If we accept the criterion of subordination, which means, if we consider symmetry groups existing in two different types within the larger type, certain types would not exist at all. For example, all the symmetry groups of the type CnK (nK) would be included in the type CnL (nL), so that the type CnK (nK) would not exist at all, and so on. A similar problem may occur with the same similarity symmetry group that can be defined by different sets of parameters n, k, q... To consequently solve that problem, it is necessary to accept the common criterion of maximal symmetry. Such an overlapping of different types of the similarity symmetry groups of rosettes S20 is possible to avoid by accepting Weyl's condition k > 0 for all the similarity symmetry groups of rosettes S20 and the condition 0 < |q| < p/n for symmetry groups of the type CnL (nL).
Cayley diagrams (Figure 3.2):