| |
|
|
| |
Volume 4, Issue 4, Article 76 |
|
|
|
|
|
| |
Certain Bounds for the Differences of Means
|
| |
|
Authors: |
Peng Gao, |
|
| |
|
Keywords:
|
Ky Fan's inequality, Levinson's inequality, Generalized weighted power means, Mean value theorem. |
|
| |
|
Date Received:
|
17/08/02 |
|
| |
|
Date Accepted:
|
03/06/03 |
|
| |
|
Subject Codes: |
26D15,26D20
|
|
| |
|
Editors: |
Kenneth B. Stolarsky, |
|
| |
|
|
|
|
|
| |
|
Abstract: |
Let 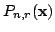 be the generalized weighted power means. We consider bounds for the differences of means in the following form: be the generalized weighted power means. We consider bounds for the differences of means in the following form: Here ![$ beta neq 0,sigma_{n,t,beta}(mathbf{ x})=sum_{i=1}^{n}omega_i[x^{beta}_i-P^{beta}_{n,t}(mathbf{x})]^2$](images/089_02/img5.gif) and and 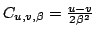 . Some similar inequalities are also considered. The results are applied to inequalities of Ky Fan's type. . Some similar inequalities are also considered. The results are applied to inequalities of Ky Fan's type.
|
|
|
|
|
|
| |
|
|
|
|
| |
|
|
|
|
|



