| |
|
|
| |
Volume 6, Issue 2, Article 28 |
|
|
|
|
|
| |
On the Inequality of P. Turan for Legendre Polynomials
|
| |
|
Authors: |
Eugen Constantinescu, |
|
| |
|
Keywords:
|
Orthogonal polynomials, Legendre polynomials, Turan Inequality, Positivity. |
|
| |
|
Date Received:
|
10/01/05 |
|
| |
|
Date Accepted:
|
03/02/05 |
|
| |
|
Subject Codes: |
33C10, 26D20.
|
|
| |
|
Editors: |
Alexandru Lupas (1942-2007), |
|
| |
|
|
|
|
|
| |
|
Abstract: |
Our aim is to prove the inequalities where 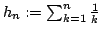 and and 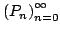 are the Legendre polynomials . At the same time, it is shown that the sequence having as general term is non-decreasing for are the Legendre polynomials . At the same time, it is shown that the sequence having as general term is non-decreasing for ![$ xin lbrack -1,1].$](images/027_05_JIPAM/img5.gif)
|
|
|
|
|
|
| |
|
|
|
|
| |
|
|
|
|
|



