| |
|
Abstract: |
Given the positive real numbers 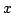 and and 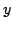 , let , let 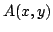 , , 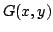 , and , and 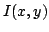 denote their arithmetic mean, geometric mean, and identric mean, respectively. It is proved that for denote their arithmetic mean, geometric mean, and identric mean, respectively. It is proved that for 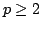 , the double inequality , the double inequality holds true for all positive real numbers 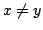 if and only if if and only if 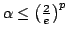 and and 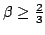 . This result complements a similar one established by H. Alzer and S.-L. Qiu [Inequalities for means in two variables, Arch. Math. (Basel) 80 (2003), 201-215]. . This result complements a similar one established by H. Alzer and S.-L. Qiu [Inequalities for means in two variables, Arch. Math. (Basel) 80 (2003), 201-215].
|



