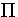 V has two
distinct critical points Q1 and Q2 near
P with
V has two
distinct critical points Q1 and Q2 near
P with
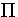 V(Q1) =
V(Q1) =
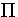 V(Q2).
For a function graph
X(x, y) = (x, y, f(x, y)),
this condition translates to N(Q1) =
(a, b) = N(Q2) and
V(Q2).
For a function graph
X(x, y) = (x, y, f(x, y)),
this condition translates to N(Q1) =
(a, b) = N(Q2) and
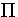 (a, b)(Q1) =
(a, b)(Q1) =
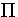 (a, b)(Q2), i.e.
(a, b)(Q2), i.e.
Property c) In terms of height functions, this condition
says that there is a unit vector V near N(P) such
that 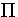 V has two
distinct critical points Q1 and Q2 near
P with
V has two
distinct critical points Q1 and Q2 near
P with
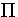 V(Q1) =
V(Q1) =
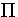 V(Q2).
For a function graph
X(x, y) = (x, y, f(x, y)),
this condition translates to N(Q1) =
(a, b) = N(Q2) and
V(Q2).
For a function graph
X(x, y) = (x, y, f(x, y)),
this condition translates to N(Q1) =
(a, b) = N(Q2) and
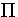 (a, b)(Q1) =
(a, b)(Q1) =
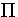 (a, b)(Q2), i.e.
(a, b)(Q2), i.e.
fx(x1, y1) =a = fx(x2, y2)
fy(x1, y1) = b = fy(x2, y2)
f(x1, y1) + ax1 + by1 = f(x2, y2) + ax2 + by2
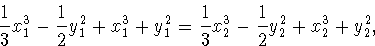
For Menn's surface we use the symmetry about the plane of the
second two coordinates. If
x1 = -x2 and
y1 = y2, then
f(x1, y1) = f(x2, y2),
fx(x1, y1) = -fx(x2, y2), and
fy(x1, y1) = fy(x2, y2)
Thus if
fx(x, y) = 0,
x 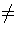 0, then
Q1 = (x, y) and
Q2 = (-x, y) will be a
pair of distinct points with the same tangent plane. Now
0 = fx(x, y) = 4
0, then
Q1 = (x, y) and
Q2 = (-x, y) will be a
pair of distinct points with the same tangent plane. Now
0 = fx(x, y) = 4 x3 + 2xy = 2x(2
x3 + 2xy = 2x(2 x2 + y) if and only if
x = 0 or y = -2
x2 + y) if and only if
x = 0 or y = -2 x2. Since this last curve
contains P = (0, 0), (c) holds for Menn's
surface. (Note that if
x2. Since this last curve
contains P = (0, 0), (c) holds for Menn's
surface. (Note that if  = -1/4,
this curve coincides with the parabolic curve, since the Gaussian
image of the parabolic curve is a single point. The same thing happens
at the top of a torus of revolution.) Theorem 3.1 (c) will be proved
in chapter 5.
= -1/4,
this curve coincides with the parabolic curve, since the Gaussian
image of the parabolic curve is a single point. The same thing happens
at the top of a torus of revolution.) Theorem 3.1 (c) will be proved
in chapter 5.
Property d) Suppose that P in U is not an umbilic point of X, i.e. X has two distinct principal curvatures k1 and k2 at P. Let V1 and V2 be unit vectors in the corresponding principal directions. Then P is a ridge point of X , with associated principal curvature k1, if the directional derivative of k1 in the direction V1 is zero at P [Por1].
Suppose that the plane g in R3 is a plane
of symmetry of the surface X . The intersection of g
with the image of X is a ridge curve of X. More
precisely if X(P) is in g, then P is
either a ridge point or an umbilic point. For let
 : (-a, a) -> U be a curve
with
: (-a, a) -> U be a curve
with  (0) = P, and let
(0) = P, and let
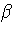 = X °
= X °  . If we choose
. If we choose
 so that r(
so that r(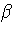 (t)) =
(t)) = 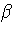 (-t), where r
is reflection in g, and t is in
(-a, a), then
(-t), where r
is reflection in g, and t is in
(-a, a), then 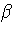 '(0) will be in a principal
direction. (
'(0) will be in a principal
direction. (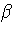 '(0) is an eigenvector of the Jacobian of the
Gauss map of X, by symmetry.) Suppose that P is not an
umbilic point, and let k be the principal curvature in the
direction of
'(0) is an eigenvector of the Jacobian of the
Gauss map of X, by symmetry.) Suppose that P is not an
umbilic point, and let k be the principal curvature in the
direction of 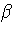 '(0). Along the curve
'(0). Along the curve 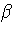 , we have
K(t) = K(-t), so
K'(0) = 0, i.e. the directional derivative of
k in the direction of
, we have
K(t) = K(-t), so
K'(0) = 0, i.e. the directional derivative of
k in the direction of 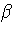 '(0) is zero, so P is a
ridge point of X. Note that for condition (d) to be satisfied,
we must also have that K(0) = 0.
'(0) is zero, so P is a
ridge point of X. Note that for condition (d) to be satisfied,
we must also have that K(0) = 0.
The shoe surface has the plane y = 0 as a plane
of symmetry, so (t, 0) is a curve of ridge points of
X. The parabolic curve is (0, t), so this ridge
crosses the parabolic curve at P = (0, 0). We
can take  (t) = (0, t), the parabolic
curve, so
(t) = (0, t), the parabolic
curve, so
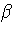 (t) = (0, t, -1/2t2),
and K(0) is the curvature of
(t) = (0, t, -1/2t2),
and K(0) is the curvature of 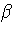 at zero, since the plane
of
at zero, since the plane
of 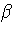 is normal to X at P. So
K(0)
is normal to X at P. So
K(0) 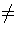 0, and (d) does not hold at P.
0, and (d) does not hold at P.
Similarly, the plane x = 0 is a plane of symmetry
of Menn's surface, so (0, t) is a ridge curve, which
crosses the parabolic curve (t,-(6
 + 1)t2) at P
= (0, 0). If we let
+ 1)t2) at P
= (0, 0). If we let
 (t) = (t, 0), then
(t) = (t, 0), then
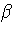 (t) = (t, 0,
(t) = (t, 0,
 t4) and
t4) and
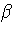 (0) = 0, so (d) does hold at P.
(0) = 0, so (d) does hold at P.
The perturbed monkey saddle has three planes of symmetry:
y = 0,
y = 31/2 x,
y = -31/2 x. So we have three
ridge curves (t, 0),
(t,31/2 t),
(t, -31/2 t). Recall that if

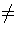 0 the parabolic curve is the circle
x2 + y2 =
0 the parabolic curve is the circle
x2 + y2 =
 2, which crosses the ridge curves at
the six points r = |
2, which crosses the ridge curves at
the six points r = | |,
|,
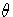 = 0,
= 0, 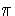 /3, 2
/3, 2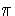 /3,
/3, 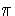 , 4
, 4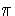 /3, 5
/3, 5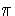 /3 in polar
coordinates. For example consider the ridge curve (t, 0)
which crosses the parabolic curve at
P1 = (
/3 in polar
coordinates. For example consider the ridge curve (t, 0)
which crosses the parabolic curve at
P1 = ( , 0)
and P2 = (-
, 0)
and P2 = (-
 , 0). Let
, 0). Let
 1(t) = (
1(t) = (
 , t), so
, t), so
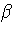 1(t) = (
1(t) = (
 , t,4
, t,4
 3/3). The curvature of
3/3). The curvature of
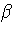 1 is zero at t = 0
(
1 is zero at t = 0
(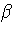 1 is a straight line) so (d) is satisfied at
P1.
Let
1 is a straight line) so (d) is satisfied at
P1.
Let  2(t) = (-
2(t) = (-
 , t), so
, t), so
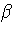 2(t) = (-
2(t) = (-
 , t, 2
, t, 2 3/3 + 2
3/3 + 2 t2), and the curvature of
t2), and the curvature of
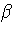 2 is zero at t = 0, so (d) is not
satisfied at P2. Since X has 3-fold symmetry
about the z-axis, we conclude that if
2 is zero at t = 0, so (d) is not
satisfied at P2. Since X has 3-fold symmetry
about the z-axis, we conclude that if
 > 0 then (d) is satisfied at
r =
> 0 then (d) is satisfied at
r =  ,
,
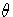 = 0, 2
= 0, 2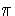 /3, 4
/3, 4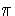 /3, and if
/3, and if
 < 0 then (d) is satisfied at
r = -
< 0 then (d) is satisfied at
r = - ,
,
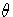 =
= 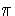 /3,
/3, 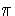 , 5
, 5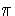 /3.
/3.
For surfaces of revolution every plane containing the z-axis is a plane of symmetry, so all the meridians are ridges. Along a circle of latitude corresponding to an inflection of the profile curve, the principal curvature associated to the meridian ridges is constant, nonzero. Along a latitude corresponding to an extremum of the profile curve the principal curvature associated to the meridian ridges is identically zero.
Finally, the warped torus has n vertical planes of symmetry, each of which intersects the parabolic curve in four points, and (d) holds at each of these points.
Theorem 3.1(d) will be proved in chapter 6.
Property e) For example, the parallel surface of a canal
surface of a space curve  is a parallel tube of
is a parallel tube of  . A
parallel tube of radius D has swallowtail singularities at
those points in space where the osculating sphere of
. A
parallel tube of radius D has swallowtail singularities at
those points in space where the osculating sphere of  has radius
D. (Cf. chapter 6 below.) Thus (e) says that the osculating
sphere of
has radius
D. (Cf. chapter 6 below.) Thus (e) says that the osculating
sphere of  has infinite radius, which occurs at the torsion
zeros of
has infinite radius, which occurs at the torsion
zeros of  . Theorem 3.1(e) will be proved in chapter 6.
. Theorem 3.1(e) will be proved in chapter 6.
Property f) If we assume that the singular locus of the pedal surface of X from the point A is a cuspidal edge with isolated swallowtail points, and a degenerate singularity at A itself, then it is easy to check that the swallowtail points of the pedal surface correspond to the cusps of the Gauss map. (This assumption is valid if the Gauss map is stable, as we shall show in chapter 6). For simplicity we assume that A is the origin of R3. The pedal map W of X from the origin is defined by
W = (X . N)N,
where N is the Gauss mapping of X. Thus
Wx = (Xx . N + X . Nx)N + (X . N)Nx,
Wy = (Xy . N + X . Ny)N + (X . N)Ny,
Wx x Wy
=
(Xx . N + X . Nx)
(X . N)
N x Ny
+
(Xy . N + X . Ny)
(X . N)
Nx x N
+
(X . N)2 Nx x Ny
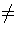 0, i.e. the tangent plane to X at
P does not pass through the origin, then
X . N
0, i.e. the tangent plane to X at
P does not pass through the origin, then
X . N 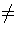 0, and
Wx x Wy = 0
if and only if
Nx x Ny = 0. Thus
the pedal map W is singular at (x, y) if and
only if the Gauss map N is singular at
(x, y), i.e. (x, y) is a parabolic
point of X . So the cuspidal edge of W corresponds to
the parabolic curve of X . Now if X(t) is the
parabolic curve, we have
0, and
Wx x Wy = 0
if and only if
Nx x Ny = 0. Thus
the pedal map W is singular at (x, y) if and
only if the Gauss map N is singular at
(x, y), i.e. (x, y) is a parabolic
point of X . So the cuspidal edge of W corresponds to
the parabolic curve of X . Now if X(t) is the
parabolic curve, we have
W(t) = (X(t) . N(t))N(t),
W'(t) = (X'(t) . N(t) +
X(t) . N'(t))N(t) +
(X(t) . N(t))N'(t)
If
X(t) . N(t) 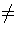 0,
then W'(t) = 0 only if N'(t) is
parallel to N(t), which can happen only if
N'(t) = 0. Thus the cusps of the cuspidal
edge W(t), which are the swallowtail points of W,
correspond to the cusps of the parabolic image N(t),
which are the cusps of the Gauss mapping N.
0,
then W'(t) = 0 only if N'(t) is
parallel to N(t), which can happen only if
N'(t) = 0. Thus the cusps of the cuspidal
edge W(t), which are the swallowtail points of W,
correspond to the cusps of the parabolic image N(t),
which are the cusps of the Gauss mapping N.
Theorem 3.1(f) will be proved in chapter 6.
Property g) The space curve  (t) has
nth order contact with the surface
G(x, y, z) = 0 at the
point
(t) has
nth order contact with the surface
G(x, y, z) = 0 at the
point  (t0) if and only if the function
G(t) = G(
(t0) if and only if the function
G(t) = G( (t)) vanishes to
order n at t0:
(t)) vanishes to
order n at t0:
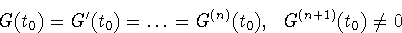
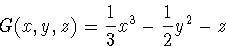
The origin (0, 0, 0) is a parabolic point, and the
tangent plane at the origin is horizontal. For the tangent line
 (t) = (t cos s, t sin s, 0),
0 < s <
(t) = (t cos s, t sin s, 0),
0 < s < 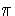 ,
G(2)(0) = -sin2 s,
G(3)(0) = 2cos3 s,
G(4)(0) = 0, so
,
G(2)(0) = -sin2 s,
G(3)(0) = 2cos3 s,
G(4)(0) = 0, so  has first order
contact with the shoe if s
has first order
contact with the shoe if s 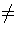 0 and second order
contact if s = 0. Next consider Menn's surface
0 and second order
contact if s = 0. Next consider Menn's surface
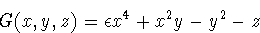
 above we have
G(2)(0) = -2 sin2 s,
G(3)(0) = 6 cos2 s sin s,
G(4)(0) = 24
above we have
G(2)(0) = -2 sin2 s,
G(3)(0) = 6 cos2 s sin s,
G(4)(0) = 24
 cos4 s,
G(5)(0) = 0. Therefore
cos4 s,
G(5)(0) = 0. Therefore  has first order contact with Menn's surface if
s
has first order contact with Menn's surface if
s 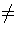 0. If
0. If

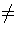 0 and s = 0 then
0 and s = 0 then
 has third order contact with the surface. If
has third order contact with the surface. If
 = 0 and s = 0 then
= 0 and s = 0 then
 has infinite order contact with the surface - in fact the
line y = z = 0 is contained in the
surface.
has infinite order contact with the surface - in fact the
line y = z = 0 is contained in the
surface.
The perturbed monkey saddle contains the three lines
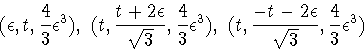
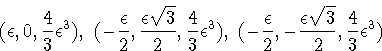
Theorem 3.1(g) will be proved in chapter 7.