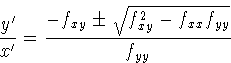
Property h) A curve (x(t), y(t)) in U is asymptotic at t if X'(t) is perpendicular to N'(t). The vector X'(t) is an asymptotic vector; its direction is an asymptotic direction. The curve is an asymptotic curve if it is asymptotic for all t. If (x(t), y(t)) is an asymptotic curve, the point (x(t0), y(t0)) is an asymptotic inflection point if the curve X(t) has curvature zero at t0.
For any curve (x(t), y(t)), X'(t) . N(t) = 0 for all t, so the curve is asymptotic at t if 0 = X'(t) . N'(t) = -X"(t) . N(t). In the case of a function graph,
X' = (x', y', fxx' + fyy')
X" = (x", y", fxx(x')2 +
2fxyx'y' +
fyy(y')2 +
fxx" + fyy")
fxx(x')2 + 2fxyx'y' + fyy(y')2
The asymptotic directions at a point are then(x', y', fxx' + fyy')
where
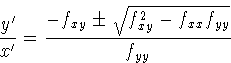
So there are 0, 1, or 2 asymptotic directions, according as the discriminant (fxy)2 - fxxfyy is negative, zero, or positive. The discriminant and the Gaussian curvature have opposite signs, so there are no asymptotic directions at an elliptic point, one at a parabolic point, and two at a hyperbolic point. The asymptotic direction at a parabolic point is the zero principal curvature direction, which is tangent to the parabolic curve at a Gaussian cusp. (Cf. (a) above).
For a generic immersion X , there are three topologically distinct configurations of asymptotic lines near a cusp of the Gauss map (see figures 3.1, 3.2, 3.3). One of these (3.1) occurs at a hyperbolic cusp, and the other two (3.2, 3.3) occur at an elliptic cusp. (Thanks to J. Callahan for pointing out an error in our original figure 3.2). This classification follows from the classification of singularities of generic multiform differential equations. (See [Lak], [A5], and also [T3], [Bro], [KeT].)
The general form for asymptotic vectors for a canal surface (example 5) is difficult to express, but it is still possible to obtain the asymptotic vectors along the parabolic curve. For a curve given by X(x(t), y(t)), we have (assuming dx/ds = 1)
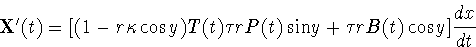
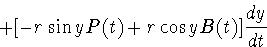
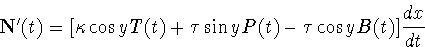
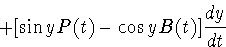

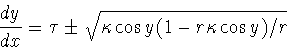
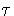 . An asymptotic
vector is then given by substituting
. An asymptotic
vector is then given by substituting
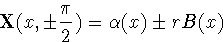
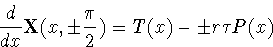

If the curve (x(t), y(t)) in
U has the property that X(t) is a straight line,
then not only is (x(t), y(t)) an
asymptotic curve, but also each point of the curve is an asymptotic
inflection point. For example, there are three such straight lines in
the perturbed monkey saddle, and these lines meet the parabolic curve
precisely at the cusps of the Gauss map (

 0). Menn's surface for
0). Menn's surface for
 = 0 also contains a straight line,
which meets the parabolic curve at the cusp of the Gauss map.
= 0 also contains a straight line,
which meets the parabolic curve at the cusp of the Gauss map.
For the case of a function graph, we can explicitly calculate the inflections of asymptotic curves. The following observation simplifies computations considerably:
If
X(x, y) = (x, y, f(x, y)),
with grad f(0, 0) = 0, and
 (t) = (t, y(t)) is a
curve in the parameter domain U, with
(t) = (t, y(t)) is a
curve in the parameter domain U, with
 (0) = 0, then the curvature of the space curve
X(t) is zero at 0 if and only if the curvature of
(0) = 0, then the curvature of the space curve
X(t) is zero at 0 if and only if the curvature of
 (t) is zero at 0 and X'(0) is an asymptotic
vector.
(t) is zero at 0 and X'(0) is an asymptotic
vector.
To prove this observation, recall that the curvature of X(t) is zero at 0 if and only if the vectors X'(0) and X"(0) are linearly dependent. We have
X'(0) = (1, y'(0), grad f(0, 0) .  '(0))
'(0))
X"(0) = (0, y"(0), grad f(0, 0) .  "(0) + D2f(0, 0)(
"(0) + D2f(0, 0)( '(0),
'(0),  '(0)))
'(0)))
 '(0),
'(0), '(0)) = 0.
Now suppose that the curvature of
'(0)) = 0.
Now suppose that the curvature of  (t) is zero at 0 and
X'(0) is asymptotic. Then
(t) is zero at 0 and
X'(0) is asymptotic. Then  "(0) = 0 and
X"(0) = 0, so the curvature of X(t) is
zero at 0. Conversely, if X"(0) = cX'(0)
then c = 0, so
"(0) = 0 and
X"(0) = 0, so the curvature of X(t) is
zero at 0. Conversely, if X"(0) = cX'(0)
then c = 0, so  "(0) = 0, hence
D2f(0, 0)(
"(0) = 0, hence
D2f(0, 0)( '(0),
'(0), '(0)) = 0.
Thus the curvature of
'(0)) = 0.
Thus the curvature of  (t) is zero at 0 and X'(0)
is asymptotic.
(t) is zero at 0 and X'(0)
is asymptotic.
Now we apply this observation to compute the asymptotic inflection points of Menn's surface. The asymptotic curves are the solution curves of the differential equation

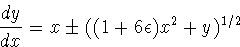
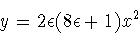
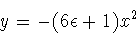

 -1/4, the asymptotic
inflection curve and the parabolic curve meet only at the origin,
where the Gauss map has a cusp.
-1/4, the asymptotic
inflection curve and the parabolic curve meet only at the origin,
where the Gauss map has a cusp.
Property i) For a curve (x(t), y(t)) with images X(t) and N(t), we have N'(0) = 0 if and only if the normal curvature KN of X(t) in the surface X(x, y) is zero at t = 0. In other words, the principal normal of X(t) is tangent to X(x, y), i.e. the osculating plane of X(t) is the tangent plane of X(x, y). If N is excellent and (x(t), y(t)) is the parabolic curve, then N'(0) = 0 if and only if P = (x(0), y(0)) is a cusp of N. So N has a cusp at P if and only if (i) holds, provided that N is excellent and the principal normal of X(t) is defined at P, i.e. the curvature of the parabolic curve X(t) is nonzero at P. This proves all but the last statement of theorem 3.2. The proof of theorem 3.2 will be completed at the end of chapter 7.
For the shoe surface or the bell surface (example 4), the parabolic curve X(t) is planar, and its plane is transverse to X(x, y), so (i) holds nowhere along the parabolic curve. For the top half of the torus of revolution (examples 4 and 5), the parabolic curve is also planar, and its plane is tangent to the torus along the entire parabolic curve. This reflects that the Gauss map is not excellent.
For Menn's surface we have the parabolic curve
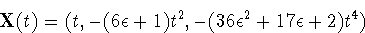

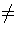 -1/6. For
-1/6. For
 = -1/6 the curvature of X(t) is
zero at t = 0, so
the above argument does not apply. (Note that in this case the
parabolic curve is planar, and its plane is orthogonal tn the tangent
plane of X(x, y) at the origin.)
= -1/6 the curvature of X(t) is
zero at t = 0, so
the above argument does not apply. (Note that in this case the
parabolic curve is planar, and its plane is orthogonal tn the tangent
plane of X(x, y) at the origin.)
If the osculating plane of the parabolic curve X(t)
equals the tangent plane of X(x, y) at
t = 0, then X(t) does not cross its
osculating plane at t = 0. It follows that if the
curvature K of X(t) is not zero at
t = 0, then the torsion T is zero at
t = 0.
For example, the torsion of the parabolic curve X(t) of Menn's surface is zero if and only if
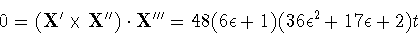
 = -1/6
and t = 0. So if 36
= -1/6
and t = 0. So if 36 2 + 17
2 + 17 + 2
+ 2 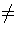 0 and
0 and

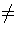 -1/6 then X(t) has zero
torsion if and only if t = 0, at the cusp of the
Gauss map. The roots of 36
-1/6 then X(t) has zero
torsion if and only if t = 0, at the cusp of the
Gauss map. The roots of 36 + 17
+ 17 + 2 = 0 are
+ 2 = 0 are
 = -1/4 and
= -1/4 and
 = -2/9; for these values of
= -2/9; for these values of
 the parabolic curve X(t) is planar. For
the parabolic curve X(t) is planar. For
 = -1/4 this plane is tangent to
X(x, y) along the entire curve
X(t), and the Gauss map is not excellent. For
= -1/4 this plane is tangent to
X(x, y) along the entire curve
X(t), and the Gauss map is not excellent. For
 = -2/9, this plane is tangent to
X(x, y) at the origin, where the Gauss map
has a cusp.
= -2/9, this plane is tangent to
X(x, y) at the origin, where the Gauss map
has a cusp.
A torsion zero of the parabolic curve of a generic surface is not necessarily a cusp of the Gauss mapping. For example the perturbed monkey saddle (example 3) has parabolic curve
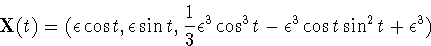

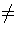 0. The torsion zeros of
X(t) occur for t = 0,
0. The torsion zeros of
X(t) occur for t = 0, 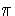 /3, 2
/3, 2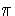 /3,
/3, 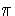 ,4
,4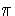 /3, 5
/3, 5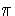 /3; but for fixed
/3; but for fixed

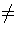 0 only half of these values occur
at cusps of the Gauss map.
0 only half of these values occur
at cusps of the Gauss map.
Property j) The asymptotic direction map of X assigns
to each point P of the parabolic curve, the line through the
origin in R3 in the asymptotic direction at
P. Locally we can choose a unit asymptotic vector field
A(P) along the parabolic curve, and the asymptotic map takes
P to A(P) in S2 . Let
(x(t), y(t)) be the parabolic curve of
X , with asymptotic image A(t) and normal image
N(t). Recall that the principal directions of X
at a point P are the eigenspaces of the Weingarten map from the
tangent space of X at P to itself. The Weingarten map is
the Jacobian of the Gauss map, followed by parallel translation. Along
the parabolic curve the Weingarten map has rank one, so its image is
the nonzero principal direction. Thus if
N'(t) 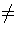 0, then N'(t) is the
nonzero principal direction. Now A(t) is in the zero principal
direction, so A(t) is orthogonal to N'(t) for all
t, i.e. A(t)-N'(t) = 0. (Since
A(t) .N(t) = 0, this is
equivalent to
A'(t) . N(t) = 0.) This
means that A(t) and N(t) are dual curves
on the sphere: N(t) is the envelope of the family of
great circles orthogonal to A(t), and vice-versa. Thus the
cusps of N(t) correspond to inflections of A(t),
and vice-versa. This implies all but the last statement of theorem
3.3. The proof of theorem 3.3 will be completed in chapter 7.
0, then N'(t) is the
nonzero principal direction. Now A(t) is in the zero principal
direction, so A(t) is orthogonal to N'(t) for all
t, i.e. A(t)-N'(t) = 0. (Since
A(t) .N(t) = 0, this is
equivalent to
A'(t) . N(t) = 0.) This
means that A(t) and N(t) are dual curves
on the sphere: N(t) is the envelope of the family of
great circles orthogonal to A(t), and vice-versa. Thus the
cusps of N(t) correspond to inflections of A(t),
and vice-versa. This implies all but the last statement of theorem
3.3. The proof of theorem 3.3 will be completed in chapter 7.
Let [x, y, z] denote the line through the origin in R3 spanned by the nonzero vector (x, y, z) (homogeneous coordinates for the projective plane). The asymptotic map of Menn's surface is
[1, t, (4 + 1)t3],
+ 1)t3],

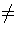 -1/4. For
-1/4. For
 = -1/4 every point of this curve is an
inflection. The normal direction along the parabolic curve is
= -1/4 every point of this curve is an
inflection. The normal direction along the parabolic curve is
[2(4 + 1)t3, -3(4
+ 1)t3, -3(4 + 1)t2, 1]
+ 1)t2, 1]
(0, 1, 3(4 + 1)t2) . (2(4
+ 1)t2) . (2(4 + 1)t3, -3(4
+ 1)t3, -3(4 + 1)t2, 1) = 0
+ 1)t2, 1) = 0
(1, t, (4 + 1)t3) . (6(4
+ 1)t3) . (6(4 + 1)t2, -6(4
+ 1)t2, -6(4 + 1)t, 1) = 0
+ 1)t, 1) = 0