| |
|
|
| |
Volume 10, Issue 4, Article 113 |
|
|
|
|
|
| |
An Extension of the Region of Variability of a Subclass of Univalent Functions
|
| |
|
Authors: |
Sukhwinder Singh, Sushma Gupta, Sukhjit Singh, |
|
| |
|
Keywords:
|
Analytic Function, Univalent function, Starlike function, Differential subordination. |
|
| |
|
Date Received:
|
03/05/2009 |
|
| |
|
Date Accepted:
|
05/11/2009 |
|
| |
|
Subject Codes: |
30C80, 30C45.
|
|
| |
|
Editors: |
Sever S. Dragomir, |
|
| |
|
|
|
|
|
| |
|
Abstract: |
We show that for ![$\alpha \in (0,2],$](images/122_09_JIPAM/img1.gif) if if 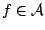 with with 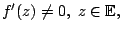 satisfies the condition satisfies the condition
then 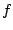 is univalent in is univalent in 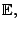 where where 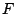 is the conformal mapping of the unit disk is the conformal mapping of the unit disk 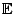 with with 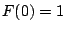 and and
Our result extends the region of variability of the differential operator
implying univalence of 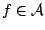 in in 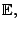 for for  . .
|
|
|
|
|
|
| |
|
|
|
|
| |
|
|
|
|
|



